We may receive a commission when you use our affiliate links. However, this does not impact our recommendations.
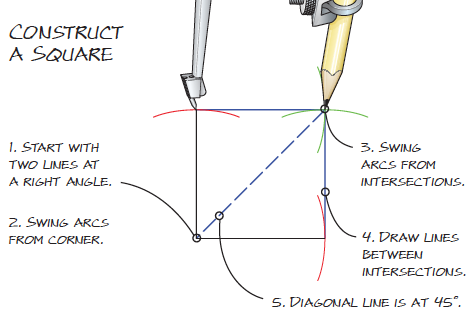
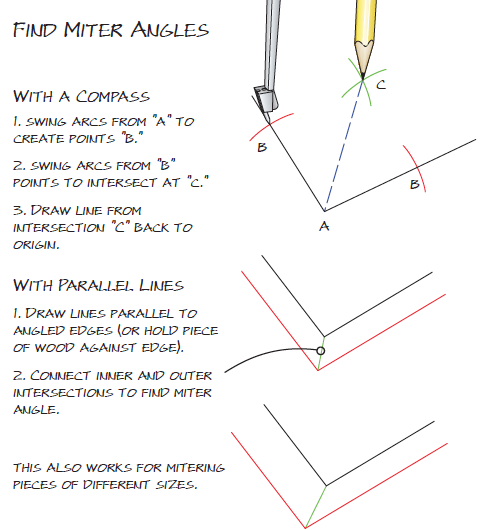
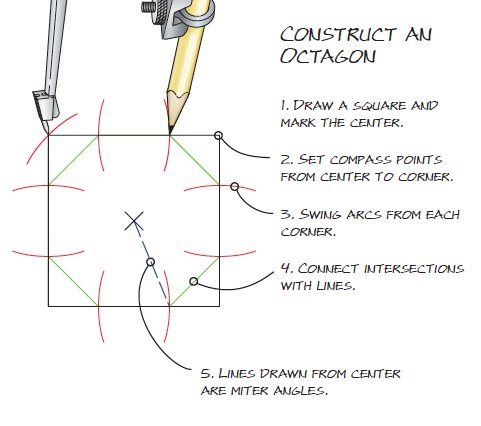
Many layout problems that seem to call for math can be quickly solved with a compass and a straightedge. Numbers can confuse and complicate the situation. What you need to know is how to make things fit –
not necessarily what numbers describe the lines and angles. Here are a few time-tested methods.
– Robert W. Lang; illustrations by Mary Jane Favorite
You may also be interested in “Essential Guide to the Steel Square” by Ken Horner.
Here are some supplies and tools we find essential in our everyday work around the shop. We may receive a commission from sales referred by our links; however, we have carefully selected these products for their usefulness and quality.












very interesting thanks
Well this was standard draftsman technique before CAD programs. If you can find a good book on drafting it will tell you how to do these and other things by just using a compass, triangles (30-60 and 45) and T-square.
The triangle/hexagon method is only approximate – there is always a gap at one corner because pi is not equal to 3, it is roughly 22/7ths. If you step the radii off from one point running around the circle you will find a noticeable gap, stepping off from 2 points reduces the visible error but there is still a gap.
You need to mark out a 60 degree section first, drawing 2 rays from the centre to the circumference and then then set the compass to the length of the chord formed where the 2 rays intersect the circumference.
Luckily a 3:4:5 ratio right angled triangle gives you 30 and 60 degreees to go with your more easily found 90.
Good idea to publish for woodworkers ~~
Thank You Mr. Lang & Ms. Jane
I enjoyed reading your return Shannonlove will look for that book.
Mr. Szczepanski~ Thats a good idea. I wonder if such an illusterated book exist ????
Working with a compass/divider is almost a lost art today prior to the late 1800s and the development of mass produced, accurately marked metal rulers and the like, everything was measured, marked and even marked using the compass.
Newton originally created the laws of gravitation using geometry and a compass, only later creating calculus to make the laws numerical. Galileo wrote a great book on using the compass to do numerical calculation. It was basically using the compass to divide lines, making a linear “gear” that could be “meshed” with other “gears” to form a on paper computer.
The compass is also the reason that the imperial/standard measuring system uses base 12 i.e. feet and inches with divisions of 2,4,8,16,32. That system is easy to use numerically with a compass while the metric system is almost impossible. The metric system didn’t really catch on until the development of mass produced marked tools.
The great advantage of using a compass is that once you master it, its the only measuring and marking tool you need. That was a great boon back in the day when everything was hauled by muscle power.
These are fantastic Robert. Thank you. Now I just need a book of these to keep by the workbench.